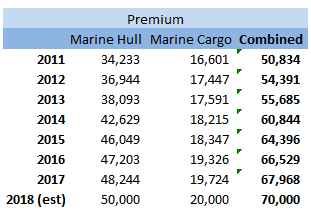
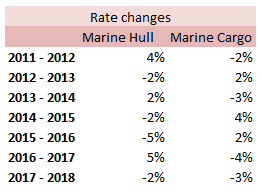
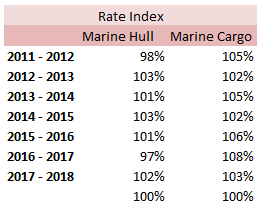
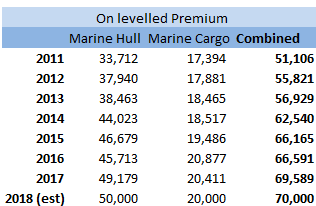
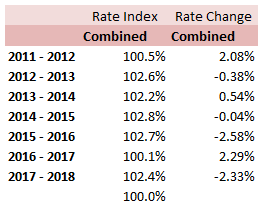
Combining two Rate Change Indices

I came across this problem at work last week, and I don't think there's anything in the notes on how to deal with it, so I have written up an explanation of how I solved it.

Suppose we are carrying out a rating exercise on a number of lines of business. For two of the lines of business, Marine Hull and Marine Cargo, we have very few claims so we have decided to group these two lines together for the purpose of the Experience Rating exercise. 

We have been supplied with separate rate change indices for all the lines of business. How do we combine the Hull and Cargo Rate Change indices?

Firstly, let's review a few basics:

What is a rate change?

It is a measure of the change in price of insurance from one year to the next when all else is constant.

Why do we need a rate change?

We will often use historic premium as an exposure measure when experience rating a book of business. If we do not adjust for the change in price of insurance then we may under or over estimate the rate of historic losses. 

What do we mean when we say the rate change 'for 2018'?
​
This answer is debatable, and if there is any uncertainty it is always better to check with whoever compiled the data, but generally the '2018' rate change means the 2017 - 2018 rate change.

How do we combine the rate changes from two lines of business?

Let's work though an example to show how to do this. I am going to be using some data I made up. These figures were generated using the random number generator in excel, please don't use them for any actual work!

Suppose we are given the following Premium estimates:

And that we are also given the following rate changes:

Then first we need to adjust the rate changes so that they are in an index. We do this by setting 2018 to be equal to 100%, and then recursively calculating the previous years using:

$${Index}_n = {Index}_{n-1} (1 - {(Rate \: Change )}_{n-1} ) $$

We can then calculate our On-Levelled Premiums, by simply multiplying our Premium figures by the Rate Change Index.

$${ ( On \: Levelled \: Premium ) }_n = ({Premium}_n) \: ({Index}_n) $$

Using the combined on-levelled premiums, we can then calculate our combined Rate Index using the following formula:

$${Index}_n = \frac{ { ( On \: Levelled \: Premium ) }_n } { {Premium}_n }  $$
And our combined Rate Change using the following formula:
$${Rate Change}_{n-1} = \frac{ {Index}_n } { {Index}_{n-1}} -1  $$

Most of the time we will only be interested in the combined On-Levelled Premium. The combined Rate Change is only a means to an end to obtain the combined On-Levelled Premium. If we have a model though where we need to input a combined Rate Change in order to group the two classes of business, then the method above can be used to obtain the required Rate Changes.